Resonance can be used to create zero impedance or zero admittance circuits at a particular resonant frequency, by either series or parallel connections of inductors and capacitors. However, care must be made not to exceed the maximum voltage or current ratings of the devices, especially in low-loss or high- circuits.
circuits.
Series Resonant Circuits
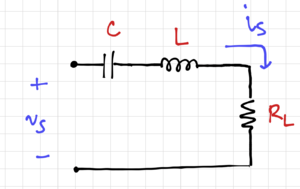
Figure 1: A series RLC circuit.
Consider the series resonant RLC circuit shown in Fig. 1. We can calculate the total impedance seen by the source  , as:
, as:
-
 |
|
(1)
|
Combining the imaginary terms of the impedance, we get:
-
 |
|
(2)
|
Resonant Frequency
We can see that the imaginary component of the impedance becomes zero at the resonant frequency,  , equal to:
, equal to:
-
 |
|
(3)
|
Note that the cancellation is narrowband, since perfect cancellation occurs only at a single frequency. Thus, from the point of view of the voltage source  , the impedance
, the impedance  is purely real, and is equal to
is purely real, and is equal to  . We can then calculate the current,
. We can then calculate the current,  as:
as:
-
 |
|
(4)
|
We then use this current to calculate the voltage across the inductor and capacitor:
-
 |
|
(5)
|
-
 |
|
(6)
|
Where  is the series circuit quality factor:
is the series circuit quality factor:
-
 |
|
(7)
|
Note that:
- For small values of
 results in large values of
results in large values of  , leading to large voltages across the inductor and capacitor.
, leading to large voltages across the inductor and capacitor.
- The voltage across the inductor is exactly
 out of phase with the capacitor voltage, thus cancelling each other out.
out of phase with the capacitor voltage, thus cancelling each other out.
Parallel Resonant Circuits
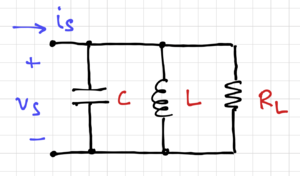
Figure 2: A parallel RLC circuit.
For the parallel resonant RLC circuit in Fig. 2, the total admittance seen by the current source,  , is:
, is:
-
 |
|
(8)
|
The admittance becomes purely real at the resonant frequency,  . Once again, we can calculate the voltage across
. Once again, we can calculate the voltage across  as:
as:
-
 |
|
(9)
|
Thus, the currents through the inductor and capacitor can be expressed as:
-
 |
|
(10)
|
-
 |
|
(11)
|
Where the parallel quality factor,  is equal to:
is equal to:
-
 |
|
(12)
|
Similar to the series resonant circuit, we see that even though the currents through the reactances cancel out, the individual currents could be very large.























