Difference between revisions of "Frequency Response Visualization"
Jump to navigation
Jump to search
| Line 1: | Line 1: | ||
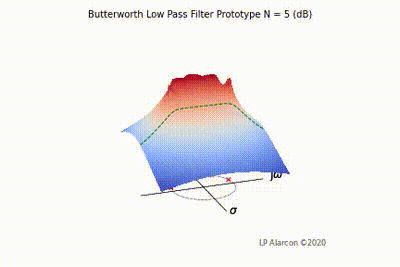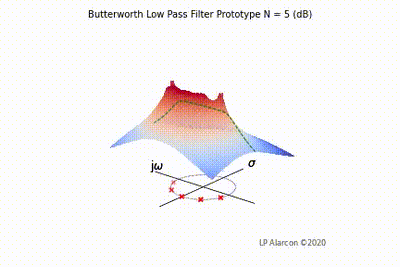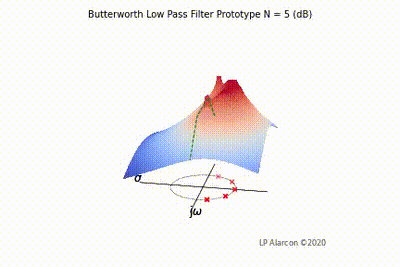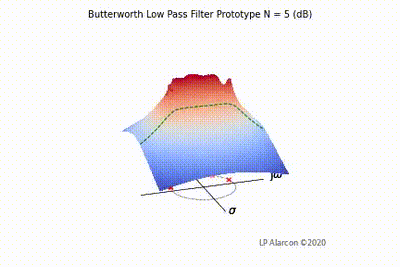
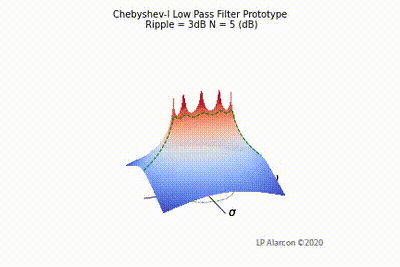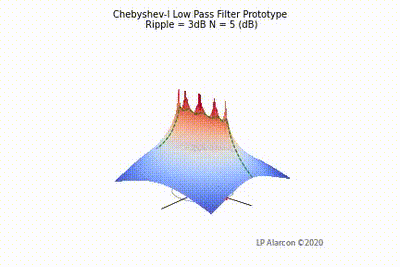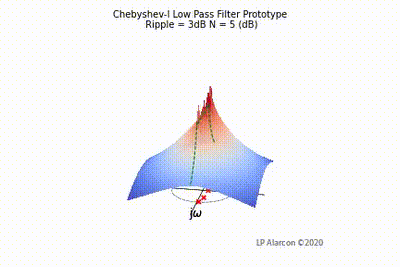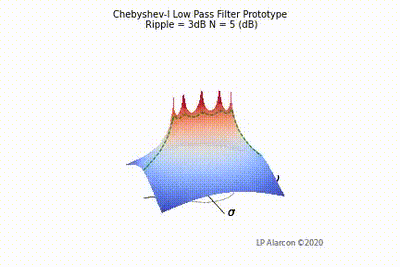
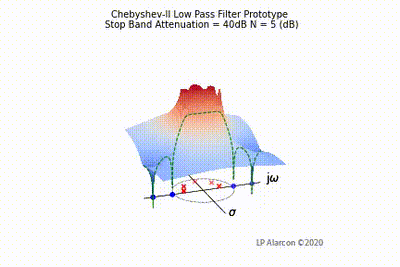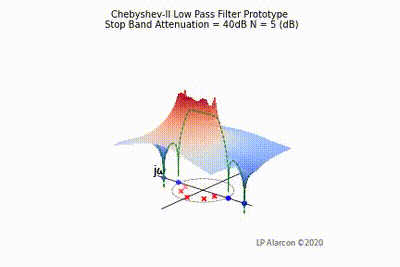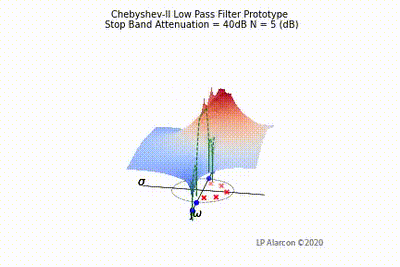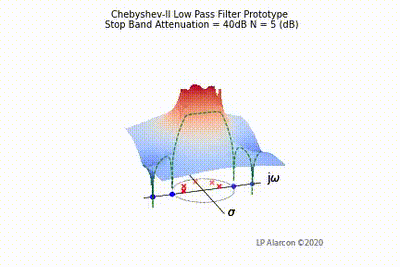
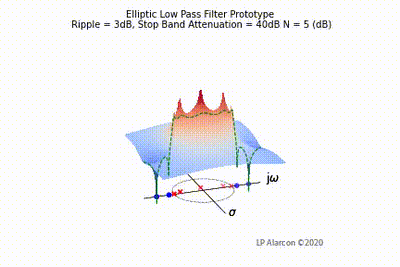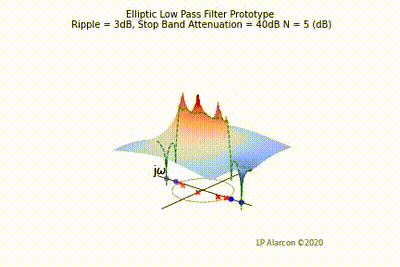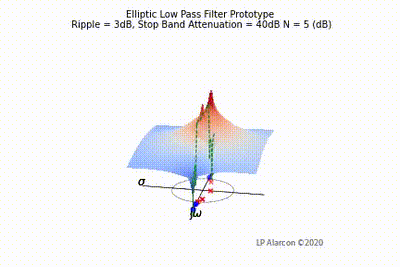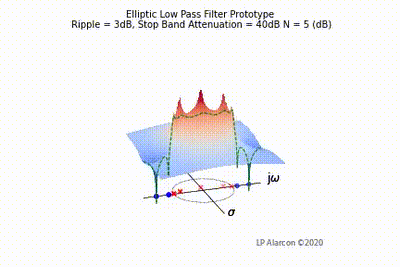
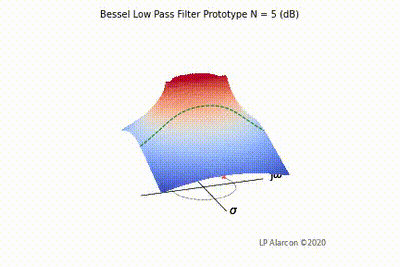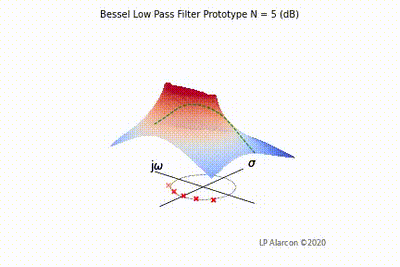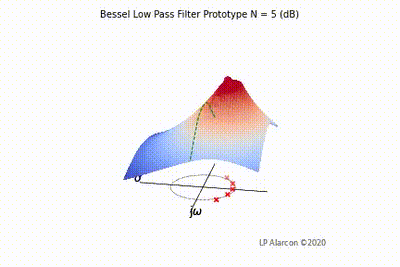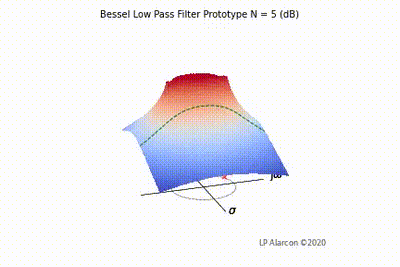
| − | The magnitude response of a linear system is the intersection of the magnitude of the transfer function, <math>\left|H\left(s\right)\right|</math>, in the <math>s=\sigma + j\omega</math>-plane and the plane containing the <math>j\omega</math>-axis, as shown in Figs. 1-4. Note that the poles are the values of <math>s</math> that makes <math>\left|H\left(s\right)\right|\rightarrow\infty</math>, and the zeros are values of <math>s</math> that result in <math>\left|H\left(s\right)\right|=0</math>. | + | The magnitude response of a linear system is the intersection of the magnitude of the transfer function, <math>\left|H\left(s\right)\right|</math>, in the <math>s=\sigma + j\omega</math>-plane and the plane containing the <math>j\omega</math>-axis, as shown by the dashed green lines in Figs. 1-4. Note that the poles are the values of <math>s</math> that makes <math>\left|H\left(s\right)\right|\rightarrow\infty</math>, and the zeros are values of <math>s</math> that result in <math>\left|H\left(s\right)\right|=0</math>. |
{| | {| | ||
Revision as of 00:15, 19 March 2021
The magnitude response of a linear system is the intersection of the magnitude of the transfer function, , in the -plane and the plane containing the -axis, as shown by the dashed green lines in Figs. 1-4. Note that the poles are the values of that makes , and the zeros are values of that result in .